Skip to content Skip to sidebar Skip to footer
Home / Change Of Base Formula, What Is The Intuitive Reasoning Behind The Change Of Base Formula In Logarithms Mathematics Stack Exchange : I could not find ways to prove this in actual practice other then through the use of the calculator.

Change Of Base Formula, What Is The Intuitive Reasoning Behind The Change Of Base Formula In Logarithms Mathematics Stack Exchange : I could not find ways to prove this in actual practice other then through the use of the calculator.

Change Of Base Formula, What Is The Intuitive Reasoning Behind The Change Of Base Formula In Logarithms Mathematics Stack Exchange : I could not find ways to prove this in actual practice other then through the use of the calculator.. The change of base formula. It's easier for us to evaluate logs of base 1 0 10 1 0 or base e e e, because calculators usually have log \log lo g and ln \ln ln buttons for these. Also see base conversion tool. We can change the base of any logarithm by using the following rule: Most calculators only accept logarithms of base 10 or base e.

Log a ( x) = log b ( x) log b ( a) log a ( x) = log b ( x) log b ( a) Does the change of base formula $$ \log_a b = \frac{\log_c b}{\log_c a} $$ have a corresponding exponent form? However, i have intentionally left one out to discuss it here in detail. Solutions to logs with various other bases located making use of graphs, or basic estimations. The change of base rule can be used if a a and b b are greater than 0 0 and not equal to 1 1, and x x is greater than 0 0.

Change Of Base Rule
Change Of Base Rule from www.chilimath.com
By base we mean how many numbers in a number system: Positive number m, log log log a b a. Using the logarithm change of base rule. Change of base formula is used in the evaluation of log and have another base than 10. This is the currently selected item. And, taking the of both sides, we get It's easier for us to evaluate logs of base 1 0 10 1 0 or base e e e, because calculators usually have log \log lo g and ln \ln ln buttons for these. The change of base formula allows us to convert a logarithm from one base to another.

1) log 3 3.3 1.087 2) log 2 30 4.907 3) log 4 5 1.161 4) log 2 2.1 1.07 5) log 3.55 0.55 6) log 6 13 1.432 7) log 6 40 2.059 8) log 4 3.5 0.904 9) log 2 2.9 1.536 10) log 6 22 1.725 11) log 7 8.7

The change of base formula is a formula for expressing a logarithm in one base in terms of logarithms in other bases. And, taking the of both sides, we get M m b = problem #1. What i want to do in this video is prove the change of base formula for logarithms change of base change of base formula which tells us write this formula formula which tells us that if i want to figure out the logarithm base a base a of x base a of x that i can figure this out by taking logarithms with a different base that this could be that this would be equal to the logarithm logarithm. The change of base rule. I'm familiar with the fact that $$ a^b = c^{b\log_c a} $$ this isn't what i'm looking for, though. The change of base formula for logarithms. On this page we look at a method to convert whole numbers and decimals to another base. \displaystyle n\ne 1 n ≠ 1. Change of base formula for logarithms. The change of base formula is an easy way to solve logarithms that have a base other than 10. Log7 (10) log 7 ( 10) rewrite log7 (10) log 7 ( 10) using the change of base formula. For any positive real numbers m, b, and n, where n ≠1 n ≠ 1 and b≠ 1 b ≠ 1, logbm =lognm lognb l o g b m = l o g n m l o g n b.

This method will work for other bases, too. Use your calculator to find the following logarithms. What i want to do in this video is prove the change of base formula for logarithms change of base change of base formula which tells us write this formula formula which tells us that if i want to figure out the logarithm base a base a of x base a of x that i can figure this out by taking logarithms with a different base that this could be that this would be equal to the logarithm logarithm. 1) log 3 3.3 1.087 2) log 2 30 4.907 3) log 4 5 1.161 4) log 2 2.1 1.07 5) log 3.55 0.55 6) log 6 13 1.432 7) log 6 40 2.059 8) log 4 3.5 0.904 9) log 2 2.9 1.536 10) log 6 22 1.725 11) log 7 8.7 A formula that allows you to rewrite a logarithm in terms of logs written with another base.

9 6 Change Of Base Formula On Vimeo
9 6 Change Of Base Formula On Vimeo from i.vimeocdn.com
\displaystyle \mathrm {ln}\left (x\right) ln(x), has base e. Use your graphing utility to graph y = log 2 (x). As always, the arguments of the logarithms must be positive and the bases of the logarithms must be positive and not equal to in order for this. By using the change of base formula, we can change a logarithmic term to allow us to input it into a calculator. The change of base formula helps to rewrite the logarithm in terms of another base log. I'm familiar with the fact that $$ a^b = c^{b\log_c a} $$ this isn't what i'm looking for, though. The change of base formula is an easy way to solve logarithms that have a base other than 10. Change of base formula we set out to prove the logarithm change of base formula:

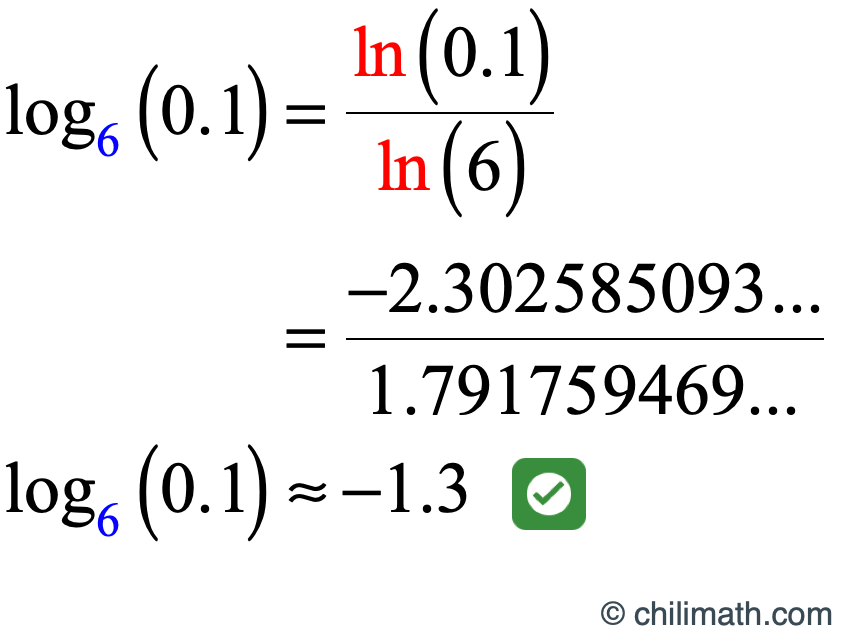
Log7 (10) log 7 ( 10) rewrite log7 (10) log 7 ( 10) using the change of base formula.

In the log change of base formula, there is no mention of exponentiation, only logarithms and division. Also see base conversion tool. Thus we have by = x. I'm familiar with the fact that $$ a^b = c^{b\log_c a} $$ this isn't what i'm looking for, though. Using the logarithm change of base rule. We take log a of each side of this equation, which. Use the change of base formula to find log base 5 of a hundred to the nearest thousandth so the change of base formula is a useful form especially when you're going to use a calculator because most calculators don't aren't don't allow you to arbitrarily change the base of your logarithm they have functions for log base e which is a natural logarithm and log base ten so you generally have to. Learn how to prove the change of base formula. Use your graphing utility to graph y = log 2 (x). What i want to do in this video is prove the change of base formula for logarithms change of base change of base formula which tells us write this formula formula which tells us that if i want to figure out the logarithm base a base a of x base a of x that i can figure this out by taking logarithms with a different base that this could be that this would be equal to the logarithm logarithm. I have discussed most of the log rules in a separate lesson. Change of base formula we set out to prove the logarithm change of base formula: This is the currently selected item.

Most calculators only accept logarithms of base 10 or base e. M m b = problem #1. Change of base log logarithm. Change of base formula is used in the evaluation of log and have another base than 10. It's easier for us to evaluate logs of base 1 0 10 1 0 or base e e e, because calculators usually have log \log lo g and ln \ln ln buttons for these.

Change Of Base Formula Logarithms Proof Video Lesson Transcript Study Com
Change Of Base Formula Logarithms Proof Video Lesson Transcript Study Com from study.com
This video explains how to use the change of base formula for logarithms to solve basic exponential equations.library: In the log change of base formula, there is no mention of exponentiation, only logarithms and division. Commonly, logarithmic equations include a base that can not be conveniently determined. This formula allows you to use your calculator, which is programmed to solve logarithms with base 10. Use your calculator to find the following logarithms. For any positive real numbers m, b, and n, where n ≠1 n ≠ 1 and b≠ 1 b ≠ 1, logbm =lognm lognb l o g b m = l o g n m l o g n b. Solutions to logs with various other bases located making use of graphs, or basic estimations. I'm familiar with the fact that $$ a^b = c^{b\log_c a} $$ this isn't what i'm looking for, though.

\displaystyle n\ne 1 n ≠ 1.

We give two examples of converting to base 26. The change of base formula date_____ period____ use a calculator to approximate each to the nearest thousandth. It's easier for us to evaluate logs of base 1 0 10 1 0 or base e e e, because calculators usually have log \log lo g and ln \ln ln buttons for these. Change of base formula is used in the evaluation of log and have another base than 10. By base we mean how many numbers in a number system: This is the currently selected item. A formula that allows you to rewrite a logarithm in terms of logs written with another base. For any positive real numbers m, b, and n, where n ≠1 n ≠ 1 and b≠ 1 b ≠ 1, logbm =lognm lognb l o g b m = l o g n m l o g n b. Use the change of base formula to find log base 5 of a hundred to the nearest thousandth so the change of base formula is a useful form especially when you're going to use a calculator because most calculators don't aren't don't allow you to arbitrarily change the base of your logarithm they have functions for log base e which is a natural logarithm and log base ten so you generally have to. Scientific calculators developed to determine logs that have a base of 10. I could not find ways to prove this in actual practice other then through the use of the calculator. Solutions to logs with various other bases located making use of graphs, or basic estimations. M m b = problem #1.

Location: